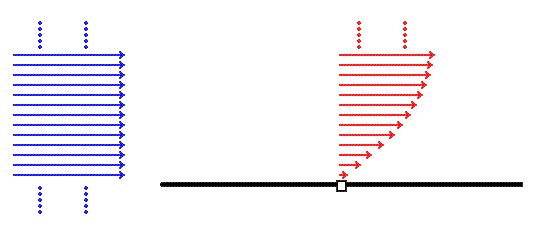
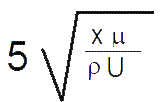Case StudiesBoundary layersIt may seem strange to call a gas a fluid but, mathematically speaking, a moving gas has many similarities with a moving liquid. The study of flowing fluids is the subject of fluid mechanics and when a fluid moves over a solid surface a boundary layer arises. Fluids have viscosity. This is readily apparent in a very sticky fluid such as honey, You can see that honey tends to stick to itself as well as to any jar from which you're trying to pour it. Air is viscous as well, though much less so than honey, and also tends to stick to itself and to any surface it is in contact with. For any fluid in contact with a solid surface, the velocity of the fluid at the fluid-solid interface is the same as that of the solid. This is called the no-slip condition. The no-slip condition has some every-day consequences for it is part of the reason why dust accumulates on fan blades. Consider the following diagram, which shows a fluid flowing over a stationary black plate aligned parallel to the fluid flow. Before it reaches the plate the fluid is flowing uniformly. The equal length blue arrows on the left indicate that throughout its depth the fluid is moving at the same velocity and the dots indicate that there's much more fluid above and below. Call the velocity indicated by the blue arrows the free-stream velocity Now consider the point on the plate marked by the white square. The red arrows show a range of velocities (or a velocity gradient) within the fluid above that particular point. The longer the arrow the greater the magnitude of the velocity. Because of the no-slip condition the fluid in contact with the plate is still but well away from the plate the fluid is moving with the free-stream velocity. In between there is a range of velocities. Very close to the plate there is a low velocity, a little further away the velocity is a little greater, a little further away still the velocity is a little greater still and so on. Above the plate each point in the fluid has its own local velocity. If you joined up the tips of the arrowheads with a line you'd get a curve that you could call the shape of the velocity gradient for the point marked with the white square. In this scenario there's nothing special about the area above the plate. Below the plate you'd have a mirror image of what's happening above the plate. Velocity gradients are associated with the term boundary layer. Steven Vogel, one of whose books is the source of much of the information on this page, has written the following in the introduction to chapter 8 of that book:
Close to a solid surface fluid viscosity has various significant effects and it would seem reasonable to differentiate such a region. But what is significant? The velocities increase gradually and continuously from zero and there is no point above the plate where viscosity effects suddenly change from significant to insignificant. There is therefore no natural upper break and it is necessary to make an arbitrary decision. The outer limit of the boundary layer is commonly defined as made up of all those points in the fluid where the local velocities are 99% of the free-stream velocity. Using this definition and making some reasonable assumptions gives the following as an approximate formula for the height of the boundary layer above the plate at a given point:
Given that X and µ are above the line, the larger these values are the greater the thickness of the boundary layer. On the other hand, since ρ and U are below the line, the larger these values are the smaller the thickness of the boundary layer. So, all other things being equal, the more viscous the fluid the thicker the boundary layer. For any given fluid and free-stream velocity, the further away from the leading edge of the plate, the thicker the boundary layer. In fact at the leading edge (where X has the value 0) the boundary layer has zero thickness. The red lines in the next diagram give you an idea of the shape of a boundary layer produced by the formula given above. The vertical distances are much exaggerated, given the size of the plate, but the diagram is qualitatively useful. You see that the boundary layer is steep near the plate's leading edge but less so downstream. The blue lines show the shapes of the velocity gradients at two points along the plate. The further downstream the 'flatter' the velocity gradient curves become. Some warningsYou'll occasionally see boundary layers referred to as areas where the air is still. As you can see that's not necessarily the case. Rather, they are zones where the effects of viscosity are significant. Strictly speaking it's even a little misleading to talk of THE boundary layer for a particular scenario. The use of the 99% factor in the definition above is very common but it is worth noting that some other definitions are in use. Depending on what viscosity-induced aspects you are studying you may find another definition far more useful for separating the 'viscous' from the 'non-viscous' regions. It's clear that the flat-plate scenario is simplistic - the real world is a much more complex place. Nevertheless the flat-plate scenario has an educational value. It allows an easy explanation of the development of velocity gradients from the no-slip condition and the derivation of a formula for the shape of a boundary layer. That in turn shows how some parameters influence boundary layers. The above discussion has been about laminar boundary layers where there is smooth flow over the surface. That's sufficient for this website. There are also turbulent boundary layers, which are much more complex. Finally, the discussion has assumed that the fluids are "everyday" fluids, such as air or water, in the various habitats that cover the earth's surface. There are more esoteric fluids (for example, very low density gases) or environments (such as very high altitudes) for which not all the above comments hold. Lichens and boundary layersBoundary layers are highly significant for small organisms that live their lives on surfaces since such organisms spend much of their time living within the boundary layers of some surface or other. For example, many crustose lichen thalli are raised very little above their substrates and so a crustose thallus is likely to lie within the substrate's boundary layer. Very slowly moving air is a good insulator and is also good at slowing the diffusion of molecules. Water that evaporates from a moist surface moves slowly through the neighbouring boundary layer, thereby prolonging the local period of humidity and so helping any nearby lichen remain hydrated and able to photosynthesize. Think of a rotting log on which some lichens are growing. The fungi that are rotting the log are breaking down complex organic molecules and releasing carbon dioxide. In the boundary layer over the log, given the slow diffusion of carbon dioxide, the carbon dioxide concentration can be much higher than that of the ambient air, giving the lichens an enhanced supply of the raw material of photosynthesis. On February 2 1989, after some days of relatively warm winter temperatures, a cold Arctic air mass that had spread southward into North America caused a rapid temperature drop to minus 25 degrees Celsius in parts of Colorado. This sudden cold change caused severe frost damage to plants and also to lichens growing on exposed boulders. At a lichen study site in Colorado it was estimated that the cooling rate could have been between 5 and 10 degrees Celsius per minute. A rate of 10 degrees per minute is fatal to most plant, animal and microbial cells. Before that rapid cooling many of the lichens on the boulders would have been metabolically active (and so more susceptible to damage) rather than dry and dormant. An investigation at the study site showed that the foliose lichens suffered badly, with many thalli being killed, but the low-growing crustose species suffered little. The foliose thalli on a boulder would have projected above the boundary layer that was warmed by heat stored in the boulder. Such severe frosts, though uncommon in the study area, could be expected to occur during the lifetimes of most lichen thalli and severe frost damage to the faster-growing foliose lichens would allow the slower-growing, but less frost-susceptible, crustose species to compete successfully on exposed boulders. Boundary layers are helpful to lichens but they also create hurdles that need to be overcome. If spores are to be wind-dispersed there's no point releasing them into the boundary layer, for they'd go virtually nowhere. It is necessary to get the spores into the turbulent or faster moving air beyond the boundary layer. In many species of apothecial or perithecial lichens the spores are ejected forcibly from the asci and shot some distance into the air, beyond the boundary layer of thallus or substrate. For example, the authors of one of the references listed below report Ramalina spores being ejected a distance of 4 to 10 millimetres, easily beyond the 1 to 2 millimetre boundary layer over an apothecium. Another way in which to help wind dispersal of propagules get beyond the boundary layer of the substrate is to produce them on raised structures. You find such raised structures on a variety of ascolichens and also in the basidiolichens. The stalked podetia of the genus Cladonia are examples from the ascolichens. In the basidiolichens think of the mushroom-like fruiting bodies produced on soil by species of Lichenomphalia. The spores are produced on the basidia that line the vertically oriented gills below the cap. Mature spores are ejected forcibly into the inter-gill airspace, move horizontally a very short distance, come to a stop and then fall down under the influence of gravity until they clear the bottom of the cap. Then, well above the soil's boundary layer the spores are able to picked up by even slight breezes and carried further afield. References
|
![An Australian Government Initiative [logo]](/images/austgovt_brown_90px.gif)